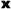|
Introduction about isosceles triangle: The isosceles(iso) triangle is one type triangle. It has three sides, in which two sides are equal in length and it has three internal angles in which two angles are equal. The following diagram shows the shape of isosceles triangle. Three sides are namely a, b and b. The two sides b are equal in length. In this article we shall discus about how to calculate area of the isosceles triangle with example problems. Formula and Example Problems: Isosceles(iso) triangle Area of isosceles(iso) triangle (A) = ½ a x h square unit. a = side length. h = height. Example problem: Find the area of isosceles(iso) triangle whose side a = 8 cm and height (h) = 12 cm. Solution: Given: a = 8 cm h = 12 cm Area of isosceles(iso) triangle (A) = ½ a x h square unit. Substitute the value of a and h in Area (A), Area (A) = ½ (8 x 12) = ½ x 96 Area (A) = 48 cm2 2. Find the area of isosceles(iso) triangle whose side a = 7 cm and height (h) = 15 cm. Solution: Given: a = 7 cm h = 15 cm Area of isosceles triangle (A) = ½ a x h square unit. Substitute the value of a and h in Area (A), Area (A) = ½ (7 x 15) = ½ x 105 Area (A) = 52.5 cm2 3. Find the area of isosceles(iso) triangle whose side a = 9 cm and height (h) = 18 cm. Solution: Given: a = 9 cm h = 18 cm Area of isosceles triangle (A) = ½ a x h square unit. Substitute the value of a and h in Area (A), Area (A) = ½ (9 x 18) = ½ x 162 Area (A) = 81 cm2 Area of Iso Triangle – Practice Problem: 1. Find the area of isosceles triangle whose side a = 5 cm and height (h) = 12 cm. Answer: Area (A) = 30 cm2 2. Find the area of isosceles triangle whose side a = 7 cm and height (h) = 16 cm. Answer: Area (A) = 56 cm2 3. Find the area of isosceles triangle whose side a = 12m and height (h) = 20m Answer: Area (A) = 120 cm2 An isosceles triangle is a triangle with (at least) two equal sides. In the figure above, the two equal sides have length b and the remaining side has length a. This property is equivalent to two angles of the triangle being equal. An isosceles triangle therefore has both two equal sides and two equal angles. The name derives from the Greek iso (same) and skelos (leg). A triangle with all sides equal is called an equilateral triangle, and a triangle with no sides equal is called a scalene triangle. An equilateral triangle is therefore a special case of an isosceles triangle having not just two, but all three sides and angles equal. Another special case of an isosceles triangle is the isosceles right triangle. The height of the isosceles triangle illustrated above can be found from the Pythagorean theorem as h=sqrt(b^2-1/4a^2). (1) The area is therefore given by A = 1/2ah (2) = 1/2asqrt(b^2-1/4a^2) (3) = 1/2a^2sqrt((b^2)/(a^2)-1/4). Learn more on aboutObtuse Triangle Definition and its Examples. Between, if you have problem on these topics Right Triangle Definition, please browse expert math related websites for more help.Please share your comment
Related Articles -
isosceles, triangle, scalene triangle,
|